Oscillations Doubt
How to identify the mean position and extreme position in these type of questions?
12 Replies
@Nimboi
Well, we can get $v=x{0}sin(t)$, so $v^{2}={x{0}}^{2}(sin^{2})=4{x{0}}^{2}(sin^{2}(t/2)(1-sin^{2}(t/2)))=4x{0}(1-x_{0})$
SirLancelotDuLac
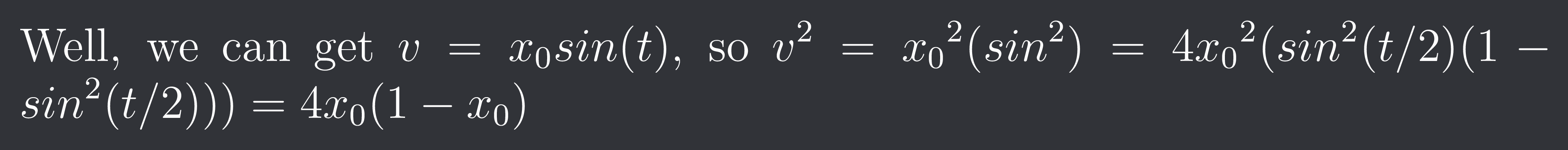
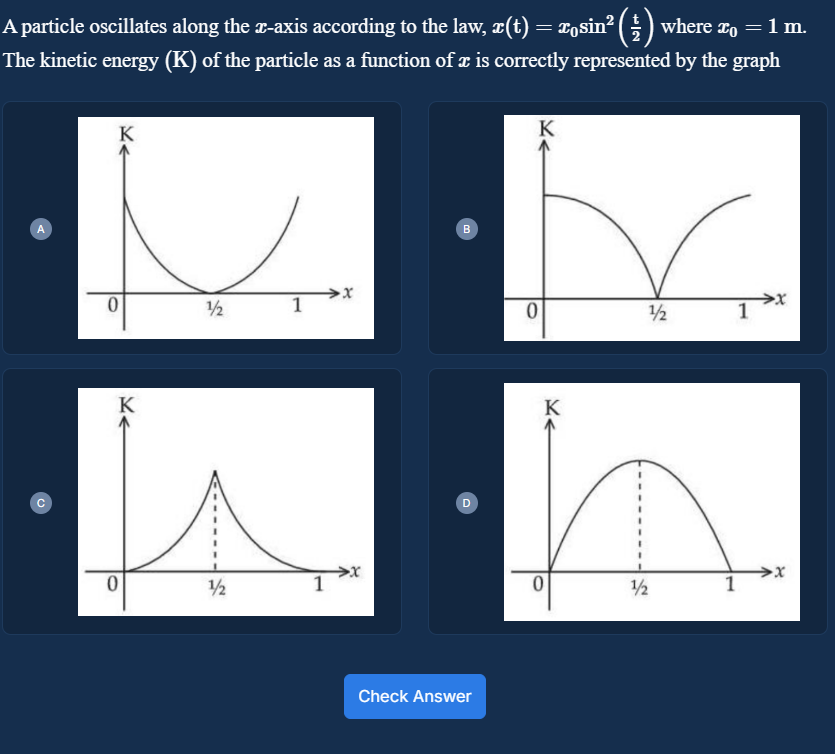
So, we can just look at the quadratic obtained and mark off d as the answer ig.
@Gyro Gearloose
@Percy what is the answer
option d
Are you able to understand this explanation
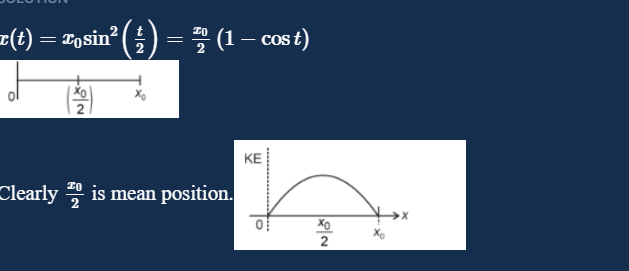
Yep. The mean position of x=a+bsin(theta) is given by a right? So basically the solution says that x/2 is the mean position and hence velocity at that point will be maximum and at x=0 and x_0 the extreme positions are obtained and hence velocity at those points are zero.
This is another way for this question.☝️
by differentiating twice you get the accn eqn and equating it to zero will give you the time at which the particle is at mean position
Thank you so much
+solved @SirLancelotDuLac @prasadam123
Post locked and archived successfully!
Archived by
<@714440046429601792> (714440046429601792)
Time
<t:1743004934:R>
Solved by
<@1075951732460376214> (1075951732460376214), <@1072150835565428826> (1072150835565428826)