field
what should be the gravitational field at the centre of hemispherical shell?
attaching my approach below
35 Replies
@Gyro Gearloose
Note for OP
+solved @user1 @user2... to close the thread when your doubt is solved. Mention the users who helped you solve the doubt. This will be added to their stats.
Its correct i believe as answer is correct
Alternatively you can assume mass to be point mass at COM of shell ie r/2 then just by formula this is obvious
correct is GM/2R^2 somehow
and why does that work ?
Physics Galaxy
YouTube
12 Physics | Electrostatics| #42 Electric Field at the Centre of Un...
PG Concept Video | Electrostatics | Electric Field at the Centre of Uniformly Charged Hemispherical Cup by Ashish Arora
Students can watch all concept videos of class 12 Electric force & electric field for jee & neet in proper sequence through PG channel playlist. Click the link below to watch all videos of electrostatics –
https://www.youtube...
Woops ig you were right its coming same after derivation
I think i mixed up something it dosent work
i believe thats what i have done
Your perpendicular distance is Rcostheta not l
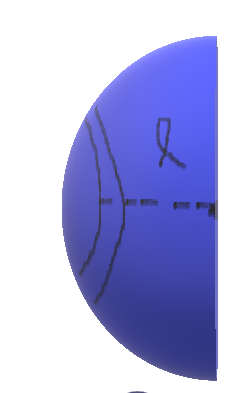
rcostheta and l are the same
l is varying
with x
See this derivation
Ask me what you do not get
ohhhh i have essentially taken r cos^2 theta
i didnt need to take component
otherwise i was doing it just fine
ill retry
Whats the problem now @SIMPle Potato

,rotate
my dm is ok right ?
dm= mass per unit area * area of element = M/2piR^2 * 2pixdx
Why is width of ur element is dx?
Its dl because of rate of change of y
Why are you even trying to complicate it in this manner trignometric substitution is there to help you just use it and integrate in terms of them
oh right its dl . regardless the result will be same since l^2=r^2-x^2 so ldl=-xdx
dm= Mxdl/r^2
dE= G* l * dm/r^3= GM x^2 dx/r^5
i didnt think of using components initially, now i just wanna know where is my method wrong/lacking
@SIMPle Potato any updates ?
I will solve it w your method and send
thanx
My results are matching with yours
I believe something is theoretically wrong proceed with trignometric proof i sent
Of PG channel
hmm thats the only possible explanation ig
btw
what will be the potential at the same time
dV= -E.dr
V = -E.dr integration
this gives V=GM/2R
however it is GM/R
@SIMPle Potato pls clarify then ill close thread
Send me the source of the question w answer
@SIMPle Potato
1.D
2.B
3.C
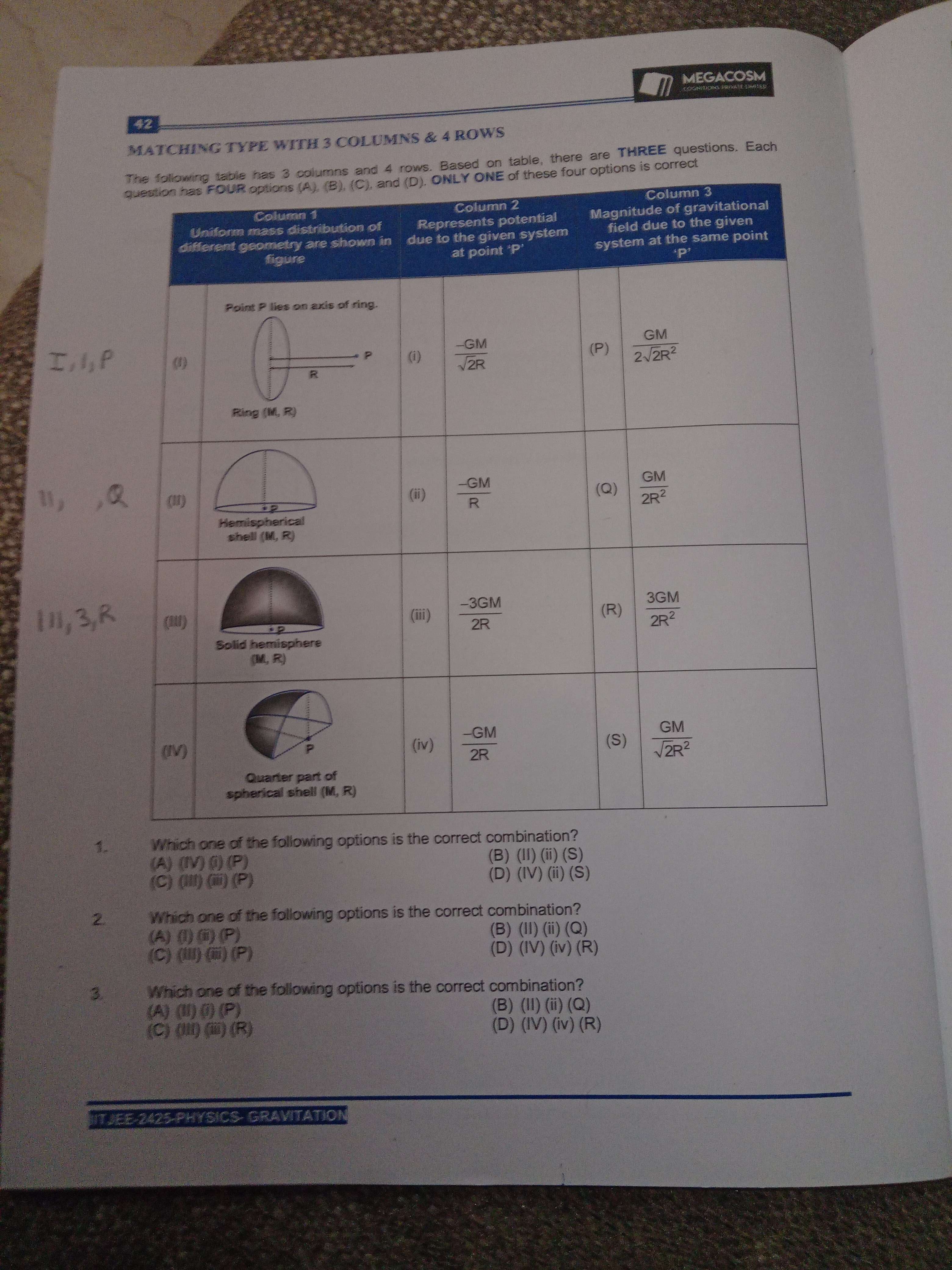
Bhai question seems out of scope for me this will involve double integration atleast to get any plaussible results
I can tell you a quick hack tho
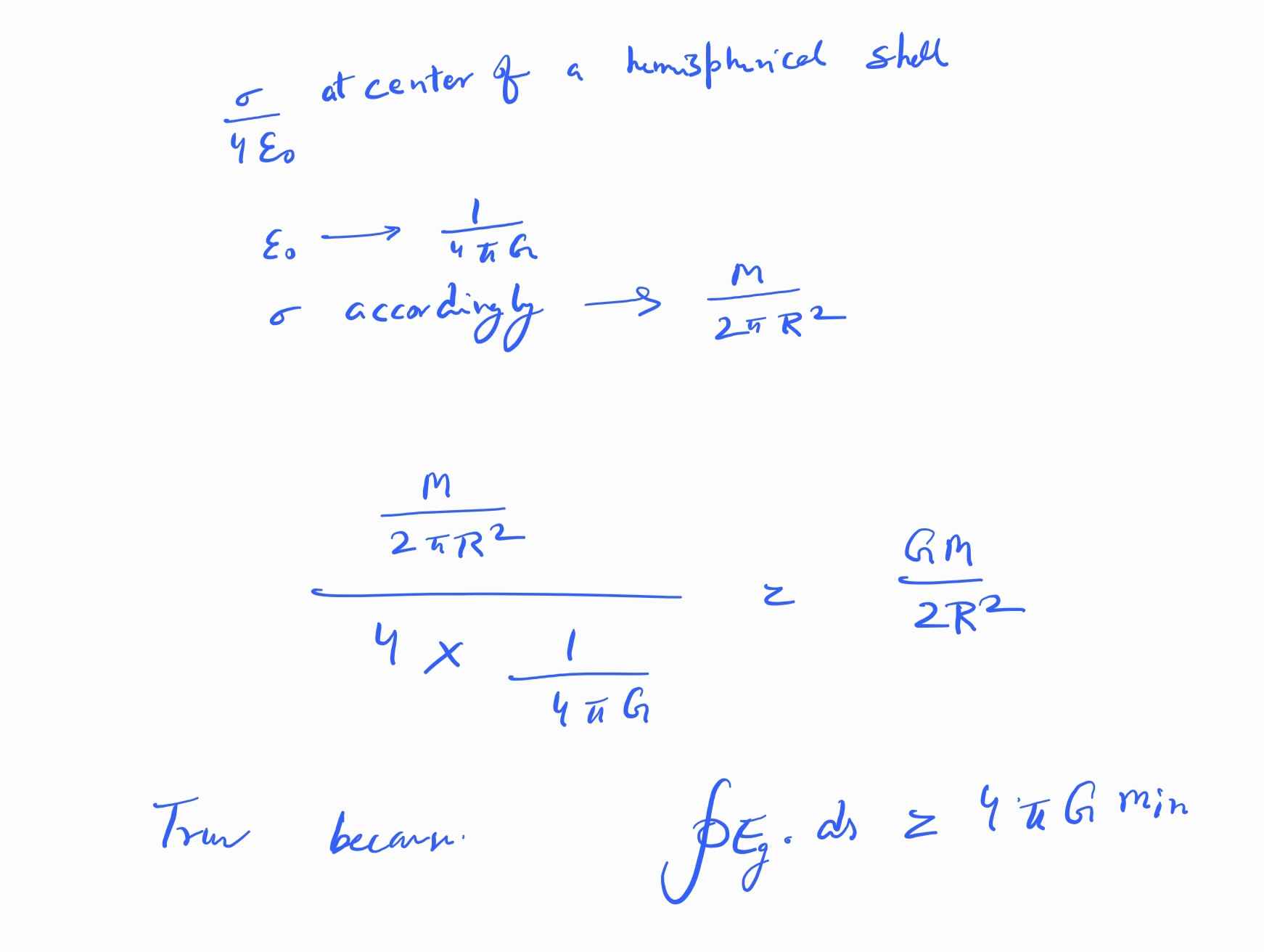
You can cross check any result this would be true
I can help only this much
Doubtnut
YouTube
Find the gravitational potential due to a hemispherical cup of mass...
Find the gravitational potential due to a hemispherical cup of mass M and radius R, at its centre of curvature.
theres exists this argument too tho
Then it should have been same for quarter sphere but it is not
no, in quarter sphere there is mass only infront of the particle not behind it
we good?
yeah
+solved @SIMPle Potato @SirLancelotDuLac
Post locked and archived successfully!
Archived by
<@741159941934415883> (741159941934415883)
Time
<t:1733067043:R>
Solved by
<@813788193286914068> (813788193286914068), <@1075951732460376214> (1075951732460376214)