application of derivatives (maxima minima)
Asked to find the least value of a€R for which 4ax²+1/x≥1, for all x>0
15 Replies
@Apu
@Apu
Note for OP
+solved @user1 @user2... to close the thread when your doubt is solved. Mention the users who helped you solve the doubt. This will be added to their stats.Note for OP
+solved @user1 @user2... to close the thread when your doubt is solved. Mention the users who helped you solve the doubt. This will be added to their stats.Now in here saying f(x)= 4ax²+1/x, if we calculate f'(1)=0 then that should work right?
As f(x)≥1 then 1 must be the point of minima as one of the roots of f'(x)?
You could go by (f'=8ax-1/x^2=0 and then find minima)
Or by AM-GM
Yeah i can put x=1 then and calculate a right as x=1 is the point of minima?l
$x=\frac{1}{2a^{\frac{1}{3}}}$, then value at minima is $4a(\frac{1}{2a^{\frac{1}{3}}})^{2}+\frac{1}{\frac{1}{2a^{\frac{1}{3}}}}$
SirLancelotDuLac
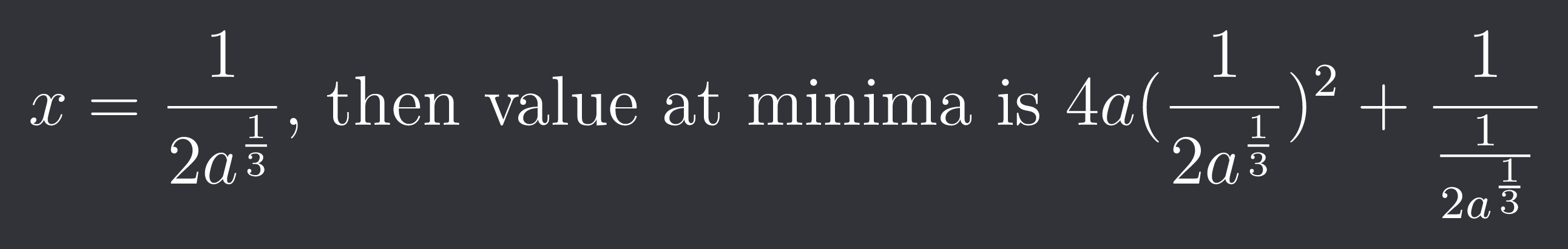
No x=1 is not minima.
why not? f(x)≥1:/?
Yeah i that part that's the value of f(1)
Ye the minimum value of f(x) is1. Not the value of x
Oh oh oh oh oh
i got it thanks
No that is the value of the minima. We put that value >=1 and then get the answer.
+solved @SirLancelotDuLac
Post locked and archived successfully!
Archived by
<@1248301167029784642> (1248301167029784642)
Time
<t:1727449909:R>
Solved by
<@1075951732460376214> (1075951732460376214)