COM
a glass shard coincides completely with the the arc of circle x^2+y^2=a^2 for all x<0 , y>0. find the the centre of mass of glass shard
42 Replies
@Gyro Gearloose
Note for OP
+solved @user1 @user2... to close the thread when your doubt is solved. Mention the users who helped you solve the doubt. This will be added to their stats.self made question so dont really know the answer
buddy glass shard ka kuch detail hai? otherwise it seems impossible
iteachchem
Transcription requested by potato jii
I think this is saying that you take the quadrant of the circle जो x less than 0 मतलब की left side में y greater than 0 मतलब उपर So that is whatever Quadrant no. 2 right If you do counter clockwise मुझे हलका सा यादे है So it must be quadrant 2 So quadrant 2 का center of mass कहा होगा I think that's what's being asked for Which should be doable
uniform mass density
to put in simpler words i want the centre of mass of a quadrant of a circle whos center is on 0,0
the arc from -a,0 to 0,a
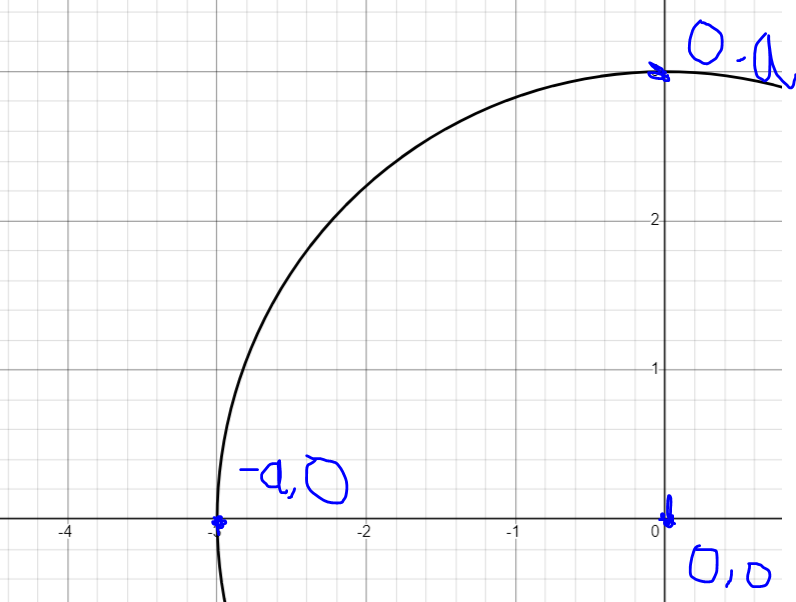
with uniform mass density
i did think of using 2rsin(theta/2)/theta/2 but i couldnt figure out the shifting of graph in that
Yo dude this is very standard formula the com lies on the bistector passing from 0,0
what if i dont know the equation of quadrant and need some general answer
What do you mean by general ans?
jaise semicircular ring hemishphere cone ka ata hai
i can try ig
(-2r/pi , 2r/pi)
this should be it ig
here the arc had angle pi/2, what bout some arbitrary angle theta ?
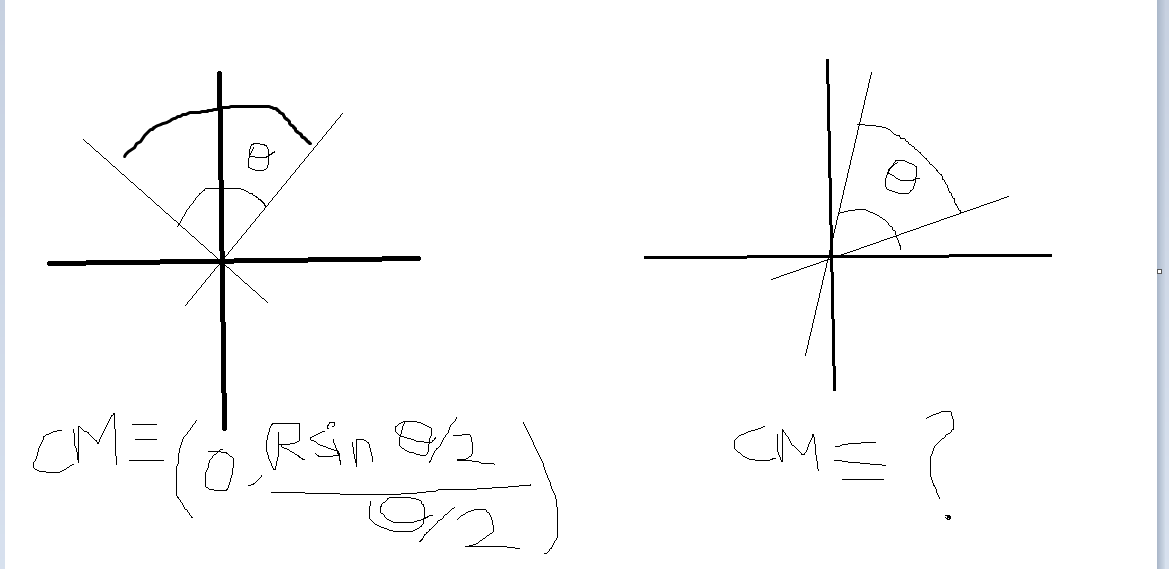
r sin theta for y and x = rcos theta
integrate from +pi/2 to pi
length of the arc is l = rthetaright yea
wahi set up the integral
how do i set that up for case 2 in the above pic
you need to know starting and end angle
arc length = r theta
so x= 1/l xtheta dl (limits theta 1 to theta2)
x = rcos theta
dl = r dtheta
change variable to theta, integrate :)
repeat for y = r sin theta
Will try
Sorry ik its a diff question but my doubt was originally a part of it so posting here
Using the above results I am getting 0,0 somehow

Com for the quad and shaded region would be the com of the square. Equate it to get the ans.
Yea use -ve mass ka idea
Got my error , thanx
2rsintheta/3theta, 2rsintheta/3theta
That's what I got someone pls verify
I think to verify integration, better to check on a site like wolfram alpha. Or some other place - even chat gpt is good.
Treatment you are good with, right?
yeah i just used centre of mass of uniform sector
Shall we close this one out?
@BANE
.
Yo ek baar yeh confirm karde
ismein ye jo tera aaya hai wo y axis pe hai i.e. the distance from intersection, 2nd case mein bhi waise hi lo bisector pe karega, vectors mein kar easy padega
I posted my answer below , verify karde
dekhta hun
.
tujhe majorly quadrant disc ka chahiye na com?
Yep
Jo rsintheta/2 / theta/2 likha hai woh arc with angle theta ka hai so don't be confused
abe wo sab chhod
Sector with angle theta ya ycm 2rsintheta/3theta hai
integrate karde naa
arc ke com ko
tune sector ka com kaise nikala like general result?
Sarthaks eConnect | Largest Online Education Community
Mass Center of a sector of a uniform circular plate Find location o...
Mass Center of a sector of a uniform circular plate Find location of mass center of a sector of a thin uniform plate.
aise
i see
+solved @iTeachChem
Post locked and archived successfully!
Archived by
<@741159941934415883> (741159941934415883)
Time
<t:1725530852:R>
Solved by
<@1035556259417571408> (1035556259417571408)