20 Replies
@Apu
Note for OP
+solved @user1 @user2... to close the thread when your doubt is solved. Mention the users who helped you solve the doubt. This will be added to their stats.@Opt
Btw what's the answer ?
Idk either. Couldn't find a solution anywhere.
Wolfram-Alpha tried but "Processing took too long."
From one of the source
I got this..@Opt

What? I don't get it. It doesn't appear in the form of a binomial term though?
Defining a series and doing recursion would probably help.
https://math.stackexchange.com/questions/1959426/prove-a-combinatoric-sum-sum-j-0k2k-j-choosej2j-frac1312
Put k = 1 and then check the options
No options for this question.
@Opt

tell me if there’s a confusion
or if the answer is wrong lol
i haven’t finished it yet, just got the recursive relationship
it should be tsub k not t sub r
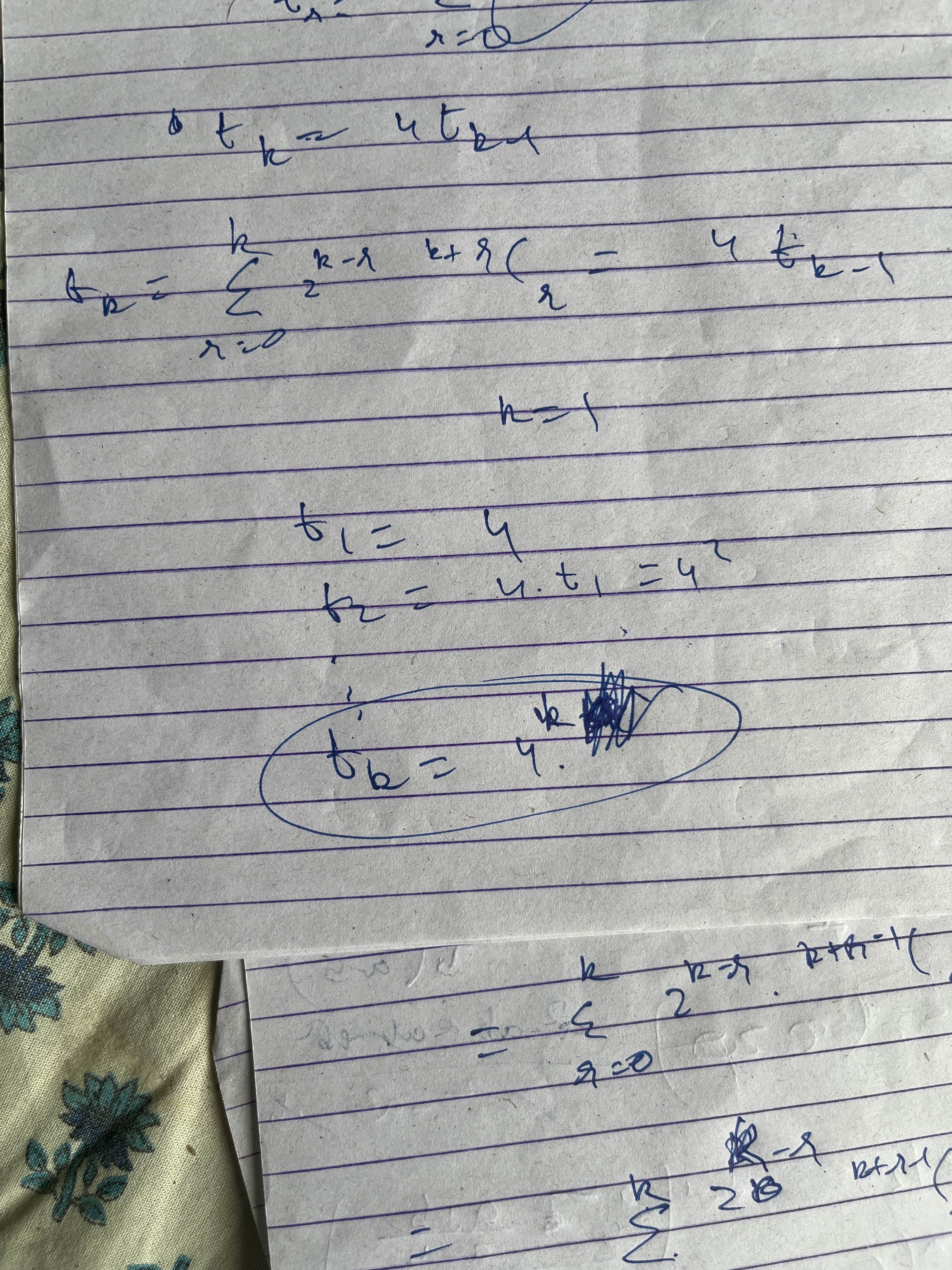
i think this is wrong!
Unfortunately I don't have the answer to this, as I got it from a test sheet, not a book.
And of course the day I say this and go on the internet for the seventh time to search (yes I kept count), I find the solution
It is indeed 4^k
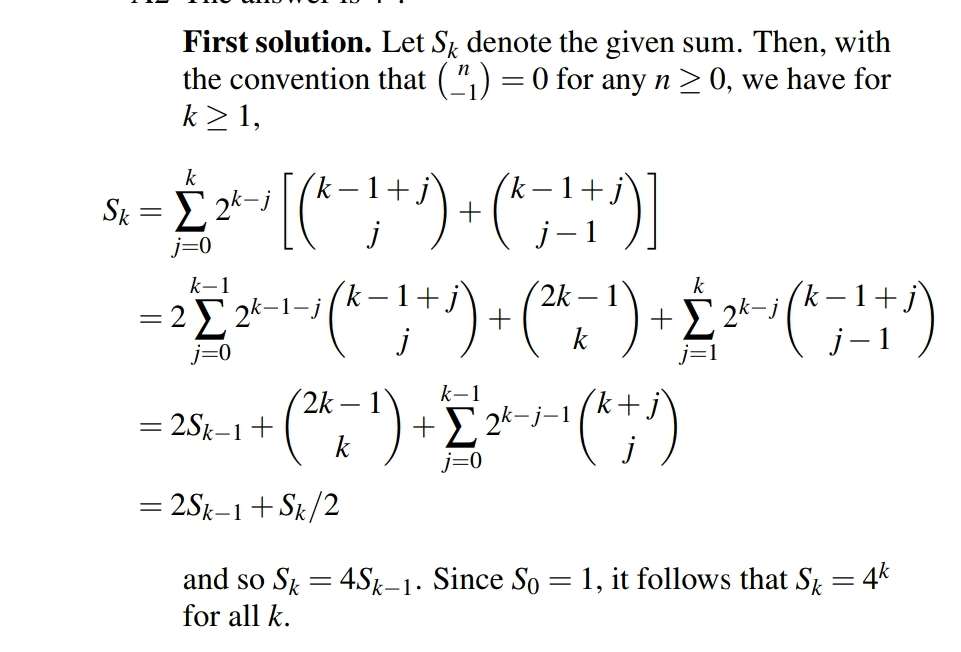
Thanks for the help
+solved @πrate
Post locked and archived successfully!
Archived by
<@763645886500175892> (763645886500175892)
Time
<t:1720179337:R>
Solved by
<@408610503376764929> (408610503376764929)
