19 Replies
@Apu
Note for OP
+solved @user1 @user2... to close the thread when your doubt is solved. Mention the users who helped you solve the doubt. This will be added to their stats.Yes
And No
It depends which infinity is bigger
Yes there are different types of infinities
Say one function was x
While other were 2x
Maybe some other were x²
.it depends on which infinity are we talking about
If the infinity above is bigger maybe it would turn out to be 0 into ♾️ form
So we can't really say that this will be the only answer
There are cases
Sometimes the answer would be a finite riyal number
Sometimes it'd be infinity large.
For example
X->♾️
(X³/3x) [2/x]
Here in the left side we have infinity by infinity while right side tends to 0
We know that the final answer is gon be 2x/3 which indeed tends to infinity
So it depends on situation
In this question we have 2 inderminant form so we cannot answer about it
firstly, you never get ∞/∞ anywhere. If the function is giving ∞ for some x, it is not defined at that point. ∞/∞ is an inderterminate form which occurs when we are studying limits. it can be removed, and the function approaches a real value, mostly.
∞/∞ * 0 can come in some limit, just solve it using the different methods we have.
secondly, ∞ * 0 is not defined. it’s like multiplying a car and a pen. absurd.
these things only appear during limits and can be handled according
it’s true that there are multiple infinities. but note that x and x^2 approach the same infinity.
the infinity of natural numbers is a different one and ‘smallest’ that we come across. it is like a countable infinity. next is the infinity of real numbers, then complex and so on
to add a bit more context, each of these is like a power set of the previous one, so size of reals is 2^(size of naturals)
hope this cleared up the confusion @Say_miracle_shadow
We may say number of natural numbers = number of whole numbers = number if integers= no. If rational numbers = number of real numbers = eleph null🫡🫡
rationals not the same size as reals
..
rationals and irrationals make up reals
Oh thank you for telling 😁
we compare sizes by bijections. i hope you know what that means ofc
if you’re able to create a bijective map between two sets, they are same size
like integers can be mapped to naturals. there is a way to map rationals as well, but with NxN
you can’t create a bijective map between rationals and reals
good discussion 😄
if the 0 is tends to 0 then the limit is still of the form of infinity/infinity
if you want proof of the statement i gave it is simply the way of solving 0*infinity form
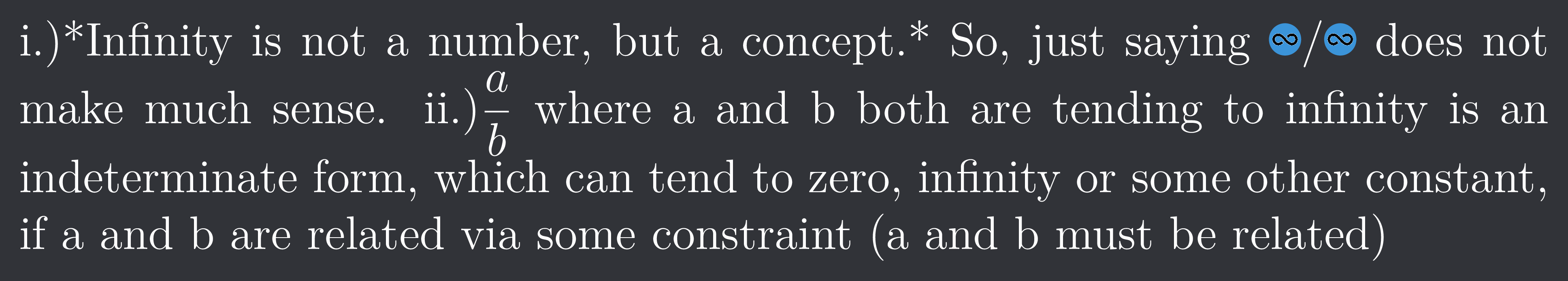
i.)Infinity is not a number, but a concept. So, just saying ♾️/♾️ does not make much sense.
ii.)$\frac{a}{b}$ where a and b both are tending to infinity is an indeterminate form, which can tend to zero, infinity or some other constant, if a and b are related via some constraint (a and b must be related)
SirLancelotDuLac
@myst1cboomer
Can you give an example of this?
Lim x--->0 xlnx
This is 0*infinity
convertible to 0/0 form, but yeah...
+ solved @Deleted User @myst1cboomer @SirLancelotDuLac @πrate @Sam
Post locked and archived successfully!
Archived by
<@1245771182104510557> (1245771182104510557)
Time
<t:1718961116:R>
Solved by
<@1221329740959318107> (1221329740959318107), <@853088063110381618> (853088063110381618), <@1075951732460376214> (1075951732460376214), <@408610503376764929> (408610503376764929), <@910910542158364672> (910910542158364672)