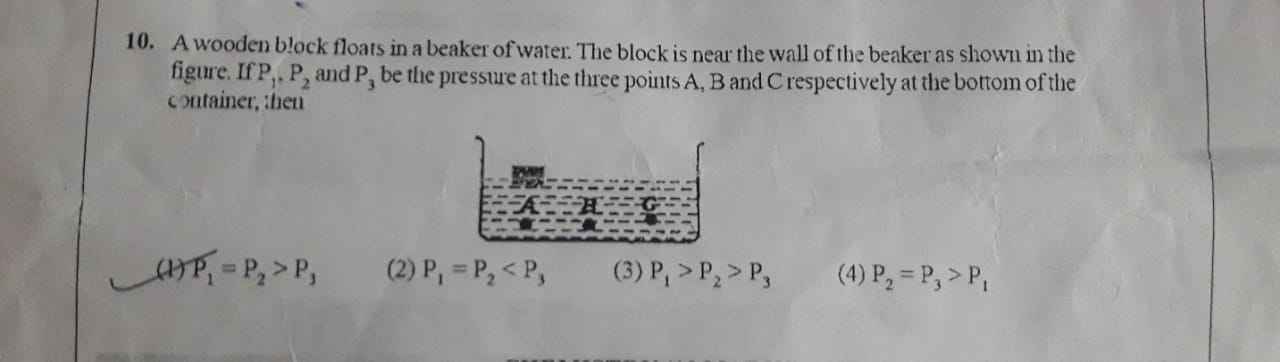
Physics doubt
Will this pressure relation remains the same when this wooden block is removed ?
23 Replies
@Gyro Gearloose
Note for OP
+solved @user1 @user2... to close the thread when your doubt is solved. Mention the users who helped you solve the doubt. This will be added to their stats.Yes as pressure at a point below height h is mass x effective g x h/volume or rogh the wooden block is there just for buyont force which is uniform in the container overall. So if u remove it, it would still be the same
Someone please correct me if I am wrong
@Optimus43 imagine two scenarios, i have a container with a piston, some air in it and water. if i press the piston, wouldnt the pressure at bottom of the water level increase?
and the total pressure we mention at depth H below the surface level is P0 + rho(g)(H), where P0 is atmospheric pressure....
so i would say if i imagine a column of water there, the pressure at A would be the normal exerted by the block on that column of water, plus the inherent mass of that column right?. so i think removing the block would change that "normal" part.
@Varun_Arora whats your opinion?
I think you are correct aswell but I think you are taking the block to be stationary there at any given time, however it can also be moving from one end to the another so can’t we just average it out?
It is moving? I thought the block was stationary i mean....🤔
Oh wait I’m sorry it’s mentioned in the question My apologies
Wait you might be right then
no worries bud, although a third confirmation would be better. i hope you got a general idea @Say_miracle_shadow
I think that shouldn't make a difference 'cause any effects by the block are being managed by buoyant forces and the system is clearly in equilibrium
I don't think the block being there makes a difference
but considering that the block is in equillibrium, would be talking about the forces on the block by the water surface right? i think pressure would be the net force the column of water exerts on the point under consideration....
a piston is a close space where moles of gas can't change, so the change occurs to keep PV=nRT valid
yup it does.....but i meant the to increase P value to increase the pressure on water level right?
ye toh sabse neeche wala element ho gaya, uske upar wale ke liye NORMAL force will be equal to the bouyont force jo uske neeche wale element par lag rha

isiliye resultant pressure rho gh hi rahega at the lower surface of the block
Fb= bouyont force
accha ruko main samajh gaya tum kya kehna chah rhe
lemme send figure
ok
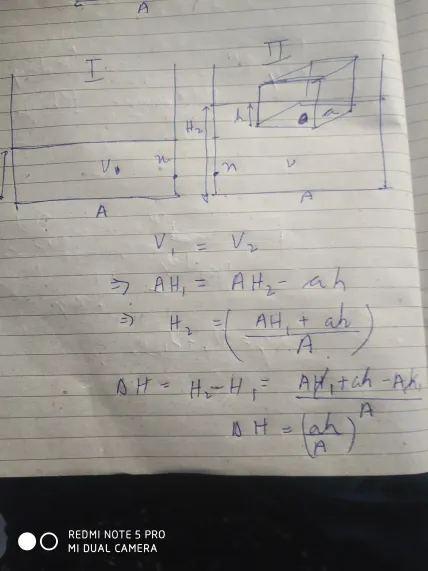
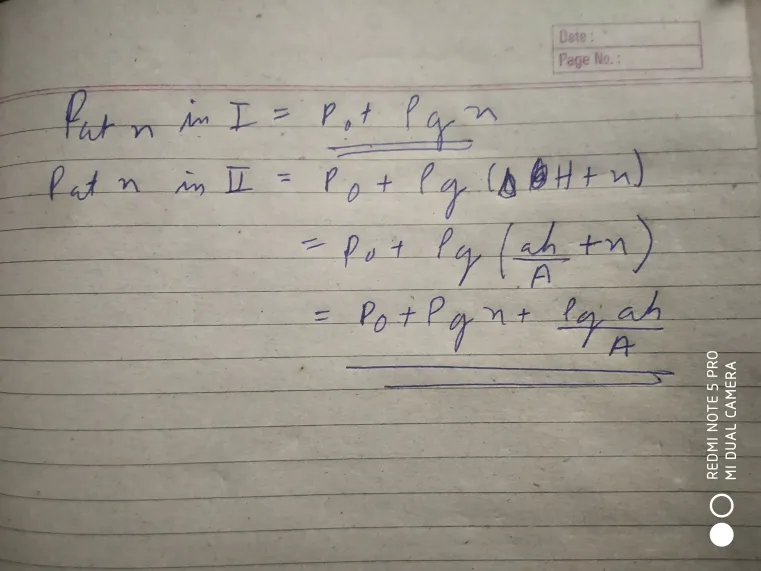
@Deleted User yahi keh rhe the na?
yup
jo normal pani ko exert karna pad rha hai uske upar rakhe block ko stable rakhne ke liye(uske weight ke barabar), utna he uss par bhi normal lag rha hai....kyunki ham sir us water column ko system consider kr rhe, toh uske upar woh N normal ka pressure ab atmosphere ke sath "N/A" extra lagega...
same agar mai pane ke container ko do alag alag atmospheric pressure wale jagahon pe rakhun like ek tumbler ko nainital ke jaun aur ek ko south mein kahin....
Block hatane se pressure is changing , right ?
P0+Pgn+Pgah/A to P0+Pgn
Is normal force by water here : buoyancy ?
Aur N/ A kis par extra lagega ?
on the water column that supports the point under consideration....
yes, suppose we marka point in the container, us point par pressure will change, but for same depth from water surface, pressure remains the same in both cases
Okay..
+solved @lmaodedXD @Deleted User @Varun_Arora @Optimus43
Post locked and archived successfully!
Archived by
<@1245771182104510557> (1245771182104510557)
Time
<t:1718715095:R>
Solved by
<@1034231350141653132> (1034231350141653132), <@1221329740959318107> (1221329740959318107), <@984016629119713290> (984016629119713290), <@886554076521840650> (886554076521840650)