how many real roots?
I saw the solution for this online and it involves finding the derivative of the equation, proving that the derivative doesn't have any real roots, which is then used to prove that the original equation must have only 1 real root. How does this work?
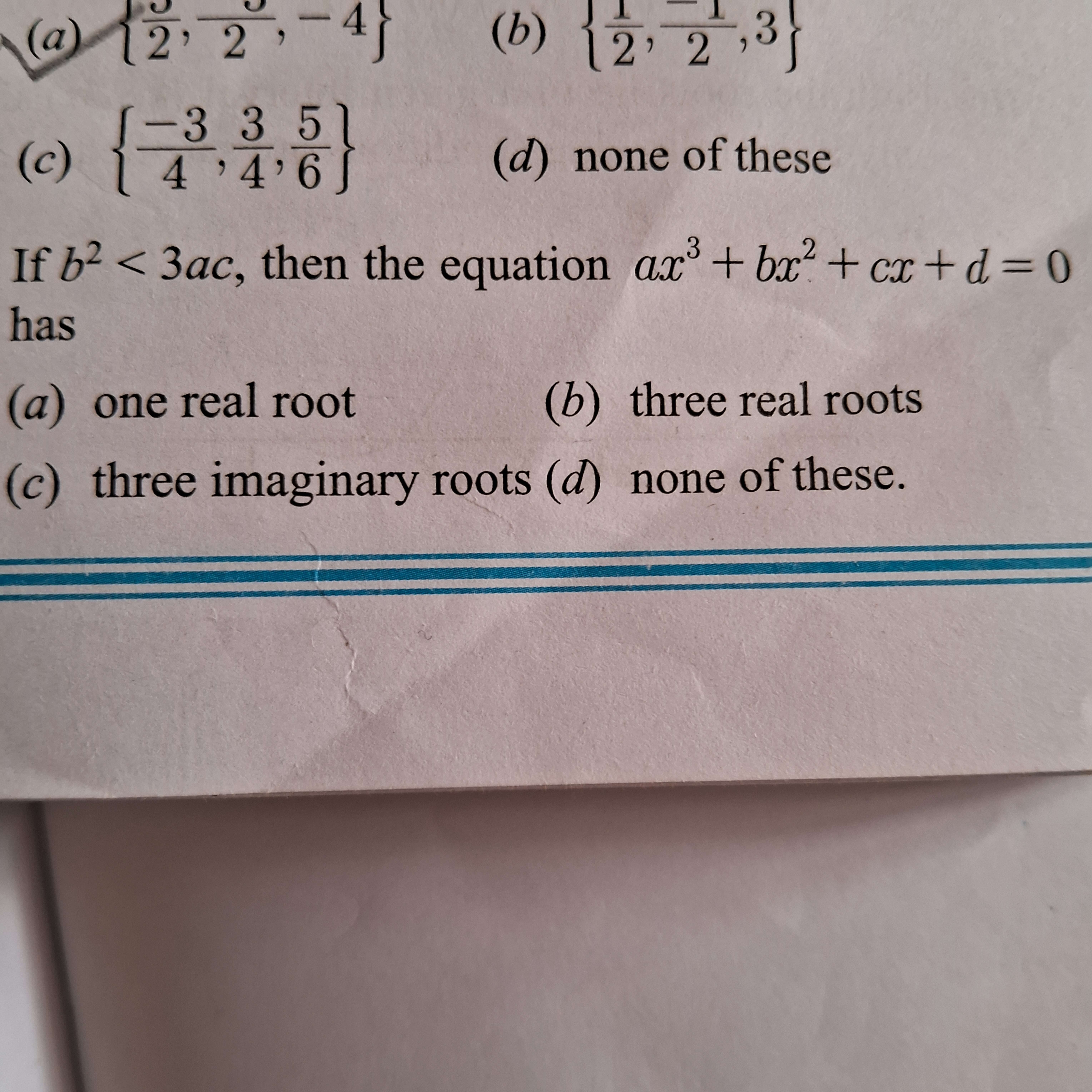
11 Replies
@Apu
Note for OP
+solved @user to close the thread when your doubt is solved. Mention the user who helped you solve the doubt. This will be added to their stats.For a polynomial function
The number of solutions decrease by 1 for the derivative provided there are no repeated roots...
E.g.
F(x) = {x-b}³{x-c}
F'(x)= 3(x-b)²(x-c) + (x-b)³
= (X-b)²[a(x)]
Ab a(x) will also have some solution
In case all the solutions were distinct..
F(x) = {x-b}{x-c}...
F'(x) =(x-c)... + (X-b)(X-d)....
Hence one solution is decreased
One more concept
If there are repeated roots for a polynomial
The derivative must have the repeated root as it's root too (other roots may change)
Thank you so much, so does it also mean that if a cubic polynomial with real coefficients has a derivative with unreal roots, then the cubic polynomial will also have unreal roots? (For solving the above the question)
Say we have a cubic polynomial w(x) = px³+qx²+rx+s where p,q,r & s belong to real numbers. Then it's derivative is w'(x) = 3px²+2qx+r
Discriminant of w'(x) is 4q²-12pr,
So, for the said polynomial to have a derivative with unreal roots, 12pr > 4q² i.e. 3pr > q²
q² is always greater than 0, so p and r must be some positive numbers which when multiplied by 3 result in a number more than q², which is possible for real numbers. That means that such a situation does exist. I don't know how would we know the nature of roots of w(x) just by knowing that 3pr > q².
thanks! will wait for a math expert to weigh in here 😄
I think I got it, since the question says 3ac > b², this matches with the condition found for the tangent being 0 at no points, (since it makes the discriminant of the derivative of the cubic function negative), the graph would be constantly increasing/decreasing, thus cutting through the x axis only once
it can have max one real root it proves that
+solved @Sam @thegamerpopz
Post locked and archived successfully!
Archived by
<@697758262186999819> (697758262186999819)
Time
<t:1715156732:R>
Solved by
<@910910542158364672> (910910542158364672)